A novel mathematical model for the design of the resonance mechanism of an intentional mistuning bladed disk system

Bladed disk systems with advanced functions are widely used in turbo-machineries. However, there are always deviations in physical dynamic properties between blades and blades due to the tolerance and wear in operation. The deviations will lead to vibration localization, which will result in high cycle fatigue and accelerate the damage of the bladed disk system. Therefore, many intentional mistuning patterns are proposed to overcome this larger local vibration. Previous studies show that intentional mistuning patterns can be used to reduce the vibration localization of the bladed disk. However, the determination of the resonance mechanism of the intentional mistuning bladed disk system is still an unsolved issue. In this paper, a novel mathematical model of resonance of an intentional mistuning bladed disk system is established. Mistuning of blades and energy resonance are included in this theoretical model. The method of the mechanical power of the rotating blade for one cycle is applied to obtain the resonance condition. By using this theoretical model, the resonance mechanism of an intentional mistuning bladed disk is demonstrated. The results suggest that the ideal results can be obtained by adjusting the intentional mistuning parameter. This paper will guide the design of the dynamic characteristics of the intentional mistuning bladed disk.

1 Introduction

Bladed disk systems are the key component for energy conversion in turbo-machinery, and it forms the largest number of components. Therefore, bladed disk systems with advanced functions are widely used. In general, in the tuned bladed disk system, the blades are designed to be identical to each other. The physical properties of each blade are universal. The vibration energy can be able to transmit uniformly in the tuned bladed disk systems. The vibration characteristics of the rotating bladed disk are complicated (Khorasany and Hutton, 2012a, b; Picou et al., 2020), and the research shows that frequency characteristics are significantly influenced by the magnitudes of forced displacements. The resonance of the tuned bladed disk satisfies specific conditions (Huang, 1981).

However, in operation, there are deviations between the blade and others due to tolerance and wear. These deviations from the physical properties of blades may lead to a larger forced vibration response in some blades (Bai et al., 2020; Li et al., 2019; Ma et al., 2016; Nikolic et al., 2007). This will result in vibration localization, and it will accelerate the high cycle fatigue of the bladed disk and the damage to the blades. Therefore, the dynamic characteristics and the energy transmission of the mistuned bladed disk are widely conducted. A dynamic model with centrifugal stiffening, Coriolis force, and other critical factors is proposed (Guo et al., 2021), and their results show that friction between adjacent shrouds can result in the complex nonlinear vibration. The altering load's effect on the vibration behaviors of cracked blades is investigated (Liu et al., 2015), and this research shows that the critical frequency is affected due to the coupling effect of the rotating speed and alternating loads. The dynamic characteristics of twisted shrouded blades are researched (Xie et al., 2017), and the mechanism of impact-caused vibration is revealed in their research. An enhanced dynamic model is proposed by considering the coupling effects of bending stretching and torsion (Yutaek et al., 2018), and their results show that the stretch variable is key for the coupling effects between stretching, bending, and torsion. The issue of the coupling effect between blade shafting and the shell is investigated in structure vibration (Liu et al., 2019), and this research shows that the natural frequency has a great influence on the acoustic radiation characteristics. The characteristics of a mistuned bladed disk with friction interfaces are researched (Pourkiaee et al., 2022; Ferhatoglu and Zucca, 2021). The coupling vibration behaviors of flexible shaft disk blades are investigated (She et al., 2018), and their result indicates that shaft and disk flexibility can influence the critical rotational speeds greatly.

In order to control the level of vibration localization of the forced response caused by mistuning, the intentional mistuning bladed disk is proposed (Han et al., 2014; Yoo et al., 2017; Martel et al., 2008; Chen et al., 2019; Beirow et al 2018, 2019). The results show that intentional mistuning has a great effect on the vibration localization. Intentional mistuning of the bladed disk is used to reduce the larger forced response (Corral et al., 2018; Martel and Sanchez-Alvarez, 2018; Gao et al., 2020; Joachim et al., 2021; Repetckii, 2020). An intentional mistuning bladed disk changes the vibration localization by designing the structure parameter (Nakos et al., 2021; Picou et al., 2018; Beirow et al., 2021; Biagiotti et al., 2018; Schlesier et al., 2018). The robust design concept is proposed to control the vibration level by the parameter design in the bladed disk systems (Chan et al., 2010). The method of using bladed packets to reduce the vibration localization of the mistuned bladed disk is researched (Kan and Zhao, 2021), and the results show that the design of the bladed packets is an alternative method to reduce the larger forced response of the mistuned bladed disk system. Di Paolo et al. (2021) introduced a friction-based passive method to reduce the vibration of a rotating structure (Di Paolo et al., 2021), and their research indicates that the dry friction can be used to reduce the vibration localization. Our previous work investigated the Coriolis effect on the intentional mistuning bladed disk. The results show that the Coriolis effect has an effect on the dynamic characteristics of the intentional mistuning bladed disk (Kan et al., 2017).

Despite the previous effort, it remains an unclear issue how to design intentional mistuning parameters in the bladed disk in theory. Therefore, the aim of this paper is to propose a theoretical model of resonance to solve this issue in the intentional mistuning bladed disk system. Mistuning of blades and energy resonance are included in this theoretical model. By using this novel model, the critical resonance mechanism of an intentional mistuning bladed disk is demonstrated. The results of this paper can provide guidance for the dynamic control of the mistuned bladed disk.

2 Model of vibration of the tuned bladed disk

In general, the bladed disk is subjected to a periodic force. This periodic force can be decomposed into a series of harmonic engine order forces. Harmonic forces are

$$\begin{array}{}\text{(1)}& f_k\left(\mathit{\theta },t\right)=f_k\sin k\left(\mathit{\omega }t+\mathit{\theta }\right),\end{array}$$

where f_k(θ,t) is the harmonic force along the circumferential direction, f_k is the amplitude of the external force of each blade, k is the engine order of the external force, ω is the frequency, and θ is the phase.

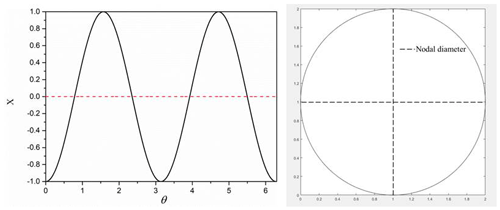
The vibration mode of the mth nodal diameter of the tuned bladed disk is depicted as

$$\begin{array}{}\text{(2)}& X_m=-A_m\cos \left({\mathit{\omega }}_{m}t+m\mathit{\theta }\right),\end{array}$$

where X_m is the mth nodal diameter and A_m is the amplitude of the mth nodal diameter. The nodal diameter is that the amplitude of vibration is zero in the bladed disk system as shown in Fig. 1. It shows the second nodal diameter of the bladed disk system in Fig. 1.

Figure 1 Sketch map of the nodal diameter.

However, there are deviations between the blade and others due to tolerance and wear. These deviations in the physical properties of blades may lead to a larger forced vibration response in some blades. This will result in vibration localization, which may accelerate the high cycle fatigue of the bladed disk and the damage to the blades.

3 Intentional mistuning of the bladed disk

An intentionally mistuned bladed disk is used to change the mistuning parameter to reduce the vibration localization of the mistuned bladed disk. Therefore, the intentional mistuning bladed disk attracts much attention. For example, harmonic intentional mistuning is one of the intentional mistuning bladed disks. Mistuning is introduced by changing Young's modulus as below (Hou and Cross, 2005; Castanier and Pierre, 2006).

$$\begin{array}{}\text{(3)}& E_i=\left(\mathrm{1}+{\mathit{\delta }}_i\right)E_{\mathrm{0}}\end{array}$$

E_i is Young's modulus of the mistuned blade. E₀ is Young's modulus of the tuned blade. For example, the harmonic intentional mistuning value of blade i is defined as

$$\begin{array}{}\text{(4)}& {\mathit{\delta }}_i=A\cos \left(\mathrm{2}\mathit{\pi }h\left(i-\mathrm{1}\right)/N\right).\end{array}$$

A is the mistuning strength, N is the total number of blades, and h is the harmonic order.

4 Novel model of the intentional mistuning bladed disk

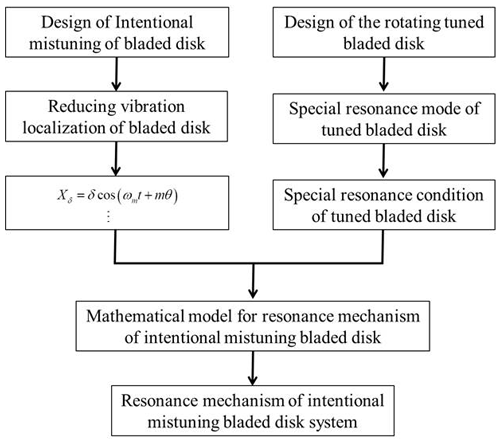
The idea of this mathematical model of the intentional mistuning bladed disk is shown in Fig. 2. First, the main dominant mode shape of the bladed system is calculated under its rated conditions. Second, the dominant mode shape obtained in the first step is brought into the theoretical model of active detuned resonance proposed in this paper. From the work done in a single cycle, according to the needs of your own working conditions, you can choose the theoretical design of your corresponding parameters.

Figure 2 Flow chart of the proposed mathematical model.

An intentional mistuning bladed disk is used to change the vibration localization by designing the structure parameter. When the blade is intentional mistuning, the mistuning can be adjusted as the design. In this paper, the vibration mode of the mth nodal diameter is depicted as

$$\begin{array}{}\text{(5)}& X_{m+\mathit{\delta }}=-\left(A_m+\mathit{\delta }\right)\cos \left({\mathit{\omega }}_{m}t+m\mathit{\theta }\right),\end{array}$$

where δ is the mistuning parameter.

When the blade goes though one period, the power of the blade is depicted:

$$\begin{array}{}\text{(6)}& W=\underset{\mathrm{0}}{\overset{\mathrm{2}\mathit{\pi }}{\int }}\underset{\mathrm{0}}{\overset{T}{\int }}{f}_k\left(\mathit{\theta },t\right){\displaystyle \frac{\partial }{\partial t}}{X}_{m+\mathit{\delta }}\left(\mathit{\theta },t\right){\displaystyle \frac{N}{\mathrm{2}\mathit{\pi }}}\mathrm{d}t\mathrm{d}\mathit{\theta },\end{array}$$

where W is the power and T is the period of the blade:

$$\begin{array}{}\text{(7)}& T={\displaystyle \frac{\mathrm{2}\mathit{\pi }}{{\mathit{\omega }}_m}}.\end{array}$$

In order to describe the energy transfer of the mistuned part, the idea of describing the energy transfer based on different parts is proposed. The power of the blade is divided into two parts, the tuning part and the mistuned part. The tuning part and the mistuned part are

$$\begin{array}{}\text{(8)}& W=W_{\mathrm{1}}+W_{\mathrm{2}},\end{array}$$

where W₁ is the power of the tuned part and W₂ is the power of the mistuned part. In this paper, the aim is to establish the bridge between the intentional bladed disk and the vibration localization. The indicators W₁ and W₂ will be able to describe the energy transfer separately.

The power of the tuned part is

$$\begin{array}{}\text{(9)}& W_{\mathrm{1}}=\underset{\mathrm{0}}{\overset{\mathrm{2}\mathit{\pi }}{\int }}\underset{\mathrm{0}}{\overset{T}{\int }}{f}_k\left(\mathit{\theta },t\right){\displaystyle \frac{\partial }{\partial t}}{X}_m\left(\mathit{\theta },t\right){\displaystyle \frac{N}{\mathrm{2}\mathit{\pi }}}\mathrm{d}t\mathrm{d}\mathit{\theta }.\end{array}$$

Taking Eqs. (1) and (2) into Eq. (9),

$$\begin{array}{}\text{(10)}& \begin{array}{l}{W}_{\mathrm{1}}=\underset{\mathrm{0}}{\overset{\mathrm{2}\mathit{\pi }}{\int }}\underset{\mathrm{0}}{\overset{T}{\int }}{f}_k\left(\mathit{\theta },t\right)\frac{\partial }{\partial t}{X}_m\left(\mathit{\theta },t\right)\frac{N}{\mathrm{2}\mathit{\pi }}\mathrm{d}t\mathrm{d}\mathit{\theta }\\ =\underset{\mathrm{0}}{\overset{\mathrm{2}\mathit{\pi }}{\int }}\underset{\mathrm{0}}{\overset{T}{\int }}{f}_k\sin \left(\mathit{\omega }t+\mathit{\theta }\right){\mathit{\omega }}_m{A}_m\sin \left({\mathit{\omega }}_{m}t+m\mathit{\theta }\right)\mathrm{d}t\mathrm{d}\mathit{\theta }\\ =-\frac{N}{\mathrm{4}\mathit{\pi }}{f}_k{\mathit{\omega }}_m{A}_m\underset{\mathrm{0}}{\overset{\mathrm{2}\mathit{\pi }}{\int }}\underset{\mathrm{0}}{\overset{T}{\int }}\left\{\cos \left[\left(k\mathit{\omega }+{\mathit{\omega }}_m\right)t+\left(k+m\right)\mathit{\theta }\right]\right.\\ -\cos \left[\left(k\mathit{\omega }-{\mathit{\omega }}_m\right)\right.\left.\left.+\left(k-m\right)\mathit{\theta }\right]\right\}\mathrm{d}t\mathrm{d}\mathit{\theta },\end{array}\end{array}$$

when

$$\begin{array}{}\text{(11)}& \left\{\begin{array}{l}{\mathit{\omega }}_m=k\mathit{\omega }\\ \text{and}\\ m=k\end{array}\right.\end{array}$$

The result of Eq. (11) is depicted as

$$\begin{array}{}\text{(12)}& \begin{array}{l}{W}_{\mathrm{1}}=-\frac{N}{\mathrm{4}\mathit{\pi }}{f}_k{\mathit{\omega }}_m{A}_m\underset{\mathrm{0}}{\overset{\mathrm{2}\mathit{\pi }}{\int }}\underset{\mathrm{0}}{\overset{T}{\int }}\left[\cos \left(\mathrm{2}{\mathit{\omega }}_{m}t+\mathrm{2}m\mathit{\theta }\right)-\mathrm{1}\right]\mathrm{d}t\mathrm{d}\mathit{\theta }\\ =-\frac{N}{\mathrm{4}\mathit{\pi }}{f}_k{\mathit{\omega }}_m{A}_m\underset{\mathrm{0}}{\overset{\mathrm{2}\mathit{\pi }}{\int }}\left\{\frac{\mathrm{1}}{\mathrm{2}{\mathit{\omega }}_m}\left[\sin \left(\mathrm{2}{\mathit{\omega }}_m\cdot \frac{\mathrm{2}\mathit{\pi }}{{\mathit{\omega }}_m}+\mathrm{2}m\mathit{\theta }\right)\right.\right.\\ \left.\left.-\sin \left(\mathrm{2}m\mathit{\theta }\right)\right]-\frac{\mathrm{2}\mathit{\pi }}{{\mathit{\omega }}_m}\right\}\mathrm{d}\mathit{\theta }=\mathit{\pi }N{f}_k{A}_m,\end{array}\end{array}$$

when

$$\begin{array}{}\text{(13)}& \left\{\begin{array}{l}{\mathit{\omega }}_m\ne k\mathit{\omega }\\ \mathrm{or}\\ m\ne k.\end{array}\right.\end{array}$$

The result of Eq. (9) is

$$\begin{array}{}\text{(14)}& \begin{array}{l}{W}_{\mathrm{1}}=-\frac{N}{\mathrm{4}\mathit{\pi }}{f}_k{\mathit{\omega }}_m{A}_m\underset{\mathrm{0}}{\overset{\mathrm{2}\mathit{\pi }}{\int }}\underset{\mathrm{0}}{\overset{T}{\int }}\left\{\cos \left[\left(k\mathit{\omega }+{\mathit{\omega }}_m\right)t+\left(k+m\right)\mathit{\theta }\right]\right.\\ \left.-\cos \left[\left(k\mathit{\omega }-{\mathit{\omega }}_m\right)+\left(k-m\right)\mathit{\theta }\right]\right\}\mathrm{d}t\mathrm{d}\mathit{\theta }\\ =-\frac{N}{\mathrm{4}\mathit{\pi }}{f}_k{\mathit{\omega }}_m{A}_m\underset{\mathrm{0}}{\overset{T}{\int }}\\ \left\{\begin{array}{l}\frac{\mathrm{1}}{k+m}\sin \left[\left(k\mathit{\omega }+{\mathit{\omega }}_m\right)t+\mathrm{2}\mathit{\pi }\left(k+m\right)\right]-\frac{\mathrm{1}}{k+m}\sin \left(k\mathit{\omega }+{\mathit{\omega }}_m\right)t\\ -\frac{\mathrm{1}}{k-m}\sin \left[\left(k\mathit{\omega }-{\mathit{\omega }}_m\right)t+\mathrm{2}\mathit{\pi }\left(k-m\right)\right]+\frac{\mathrm{1}}{k-m}\sin \left(k\mathit{\omega }-{\mathit{\omega }}_m\right)t\end{array}\right\}\mathrm{d}t\\ =\mathrm{0}.\end{array}\end{array}$$

The power of the intentional mistuning part is depicted as

$$\begin{array}{}\text{(15)}& W_{\mathrm{2}}=\underset{\mathrm{0}}{\overset{\mathrm{2}\mathit{\pi }}{\int }}\underset{\mathrm{0}}{\overset{T}{\int }}{f}_k\left(\mathit{\theta },t\right){\displaystyle \frac{\partial }{\partial t}}{X}_{\mathit{\delta }}\left(\mathit{\theta },t\right){\displaystyle \frac{N}{\mathrm{2}\mathit{\pi }}}\mathrm{d}t\mathrm{d}\mathit{\theta }.\end{array}$$

Substituting Eqs. (1) and (2) into Eq. (15), the power of the mistuned part is depicted as

$$\begin{array}{}\text{(16)}& \begin{array}{l}{W}_{\mathrm{2}}=\underset{\mathrm{0}}{\overset{\mathrm{2}\mathit{\pi }}{\int }}\underset{\mathrm{0}}{\overset{T}{\int }}{f}_k\left(\mathit{\theta },t\right)\frac{\partial }{\partial t}{X}_{\mathit{\delta }}\left(\mathit{\theta },t\right)\frac{N}{\mathrm{2}\mathit{\pi }}\mathrm{d}t\mathrm{d}\mathit{\theta }\\ =\underset{\mathrm{0}}{\overset{\mathrm{2}\mathit{\pi }}{\int }}\underset{\mathrm{0}}{\overset{T}{\int }}{f}_k\sin \left(\mathit{\omega }t+\mathit{\theta }\right){\mathit{\omega }}_m\mathit{\delta }\sin \left({\mathit{\omega }}_{m}t+m\mathit{\theta }\right)\mathrm{d}t\mathrm{d}\mathit{\theta }\\ =-\frac{N}{\mathrm{4}\mathit{\pi }}{f}_k{\mathit{\omega }}_m\mathit{\delta }\underset{\mathrm{0}}{\overset{\mathrm{2}\mathit{\pi }}{\int }}\underset{\mathrm{0}}{\overset{T}{\int }}\left\{\cos \left[\left(k\mathit{\omega }+{\mathit{\omega }}_m\right)t+\left(k+m\right)\mathit{\theta }\right]\right.\\ \left.-\cos \left[\left(k\mathit{\omega }-{\mathit{\omega }}_m\right)+\left(k-m\right)\mathit{\theta }\right]\right\}\mathrm{d}t\mathrm{d}\mathit{\theta },\end{array}\end{array}$$

when

$$\begin{array}{}\text{(17)}& \left\{\begin{array}{l}{\mathit{\omega }}_m=k\mathit{\omega }\\ \text{and}\\ m=k.\end{array}\right.\end{array}$$

The power of the intentional mistuning part is obtained. The result of Eq. (16) is

$$\begin{array}{}\text{(18)}& \begin{array}{l}{W}_{\mathrm{2}}=-\frac{N}{\mathrm{4}\mathit{\pi }}{f}_k{\mathit{\omega }}_m\mathit{\delta }{A}_m\underset{\mathrm{0}}{\overset{\mathrm{2}\mathit{\pi }}{\int }}\underset{\mathrm{0}}{\overset{T}{\int }}\left[\cos \left(\mathrm{2}{\mathit{\omega }}_{m}t+\mathrm{2}m\mathit{\theta }\right)-\mathrm{1}\right]\mathrm{d}t\mathrm{d}\mathit{\theta }\\ =-\frac{N}{\mathrm{4}\mathit{\pi }}{f}_k{\mathit{\omega }}_m\mathit{\delta }{A}_m\underset{\mathrm{0}}{\overset{\mathrm{2}\mathit{\pi }}{\int }}\left\{\frac{\mathrm{1}}{\mathrm{2}{\mathit{\omega }}_m}\left[\sin \left(\mathrm{2}{\mathit{\omega }}_m\cdot \frac{\mathrm{2}\mathit{\pi }}{{\mathit{\omega }}_m}+\mathrm{2}m\mathit{\theta }\right)\right.\right.\\ \left.\left.-\sin \left(\mathrm{2}m\mathit{\theta }\right)\right]-\frac{\mathrm{2}\mathit{\pi }}{{\mathit{\omega }}_m}\right\}\mathrm{d}\mathit{\theta }\\ =\mathit{\pi }N{f}_k\mathit{\delta },\end{array}\end{array}$$

when

$$\begin{array}{}\text{(19)}& \left\{\begin{array}{l}{\mathit{\omega }}_m\ne k\mathit{\omega }\\ \mathrm{or}\\ m\ne k.\end{array}\right.\end{array}$$

The power of the intentional mistuning part is obtained. The result of Eq. (16) is

$$\begin{array}{}\text{(20)}& \begin{array}{l}{W}_{\mathrm{2}}=-\frac{N}{\mathrm{4}\mathit{\pi }}{f}_k{\mathit{\omega }}_m\mathit{\delta }{A}_m\underset{\mathrm{0}}{\overset{\mathrm{2}\mathit{\pi }}{\int }}\underset{\mathrm{0}}{\overset{T}{\int }}\left\{\cos \left[\left(k\mathit{\omega }+{\mathit{\omega }}_m\right)t\right.\right.\\ \left.\left.+\left(k+m\right)\mathit{\theta }\right]-\cos \left[\left(k\mathit{\omega }-{\mathit{\omega }}_m\right)+\left(k-m\right)\mathit{\theta }\right]\right\}\mathrm{d}t\mathrm{d}\mathit{\theta }\\ =-\frac{N}{\mathrm{4}\mathit{\pi }}{f}_k{\mathit{\omega }}_m\mathit{\delta }\underset{\mathrm{0}}{\overset{T}{\int }}\\ \left\{\begin{array}{l}\frac{\mathrm{1}}{k+m}\sin \left[\left(k\mathit{\omega }+{\mathit{\omega }}_m\right)t\right.\\ \left.+\mathrm{2}\mathit{\pi }\left(k+m\right)\right]-\frac{\mathrm{1}}{k+m}\sin \left(k\mathit{\omega }+{\mathit{\omega }}_m\right)t\\ -\frac{\mathrm{1}}{k-m}\sin \left[\left(k\mathit{\omega }-{\mathit{\omega }}_m\right)t+\mathrm{2}\mathit{\pi }\left(k-m\right)\right]\\ +\frac{\mathrm{1}}{k-m}\sin \left(k\mathit{\omega }-{\mathit{\omega }}_m\right)t\end{array}\right\}\mathrm{d}t\\ =\mathrm{0}.\end{array}\end{array}$$

The power of the tuned and intentional mistuning parts is obtained in different situations. Summing up the above,

$$\begin{array}{}\text{(21)}& \begin{array}{l}W=\underset{\mathrm{0}}{\overset{\mathrm{2}\mathit{\pi }}{\int }}\underset{\mathrm{0}}{\overset{T}{\int }}{f}_k\left(\mathit{\theta },t\right)\frac{\partial }{\partial t}{X}_{m+\mathit{\delta }}\left(\mathit{\theta },t\right)\frac{N}{\mathrm{2}\mathit{\pi }}\mathrm{d}t\mathrm{d}\mathit{\theta }\\ =W_{\mathrm{1}}+W_{\mathrm{2}}\\ =\left\{\begin{array}{l}\mathit{\pi }N{f}_k{A}_m+\mathit{\pi }N{f}_k\mathit{\delta },{\mathit{\omega }}_m=k\mathit{\omega }\text{and}k=m\\ \mathrm{0},{\mathit{\omega }}_m\ne k\mathit{\omega }\text{or}k\ne m.\end{array}\right.\end{array}\end{array}$$

From Eq. (21), the different situations can be able to produce a different result. We can adjust the different mistuning parameter δ, and we will obtain an ideal result, as the designer expects.

5 Results and discussions

In our paper, the proposed mathematical model is not applied to all bladed disk systems. From Eqs. (9) and (15) we can see that friction is not considered in this proposed mathematical model of the bladed disk system. The friction will produce some power in the bladed disk system, when the bladed disk system contains friction. Some blade disk systems do have friction, and the blades are connected with each other through a shroud or shoulder. In this way, there is friction at the interface. Friction is very complex. Friction is related to the roughness, contact area, contact angle and positive contact pressure of the interface. When the blade is out of tune, the friction of each interface is different, and the work done by the friction is also different. Therefore, this mathematical model is not applied to bladed disks with friction.

Bladed disk systems are the key component for energy conversion in turbo-machineries. The power is important for the design of the bladed disk system. From the power of the blade, it shows that we can adjust a different mistuning parameter δ, and we will obtain an ideal result, as the designer expects.

$$\begin{array}{}\text{(22)}& \begin{array}{l}W=\underset{\mathrm{0}}{\overset{\mathrm{2}\mathit{\pi }}{\int }}\underset{\mathrm{0}}{\overset{T}{\int }}{f}_k\left(\mathit{\theta },t\right)\frac{\partial }{\partial t}{X}_{m+\mathit{\delta }}\left(\mathit{\theta },t\right)\frac{N}{\mathrm{2}\mathit{\pi }}\mathrm{d}t\mathrm{d}\mathit{\theta }\\ =\left\{\begin{array}{l}\mathit{\pi }N{f}_k{A}_m+\mathit{\pi }N{f}_k\mathit{\delta },{\mathit{\omega }}_m=k\mathit{\omega }\text{and}k=m\\ \mathrm{0},{\mathit{\omega }}_m\ne k\mathit{\omega }\text{or}k\ne m,\end{array}\right.\end{array}\end{array}$$

when the mistuning parameter is

$$\begin{array}{}\text{(23)}& \mathit{\delta }<\mathrm{0}.\end{array}$$

In this paper, the aim is to adjust the mistuning parameters to control the vibration localization of the mistuned bladed disk. Based on the proposed mathematical model, the power will be less than the tuned bladed disk system, and this illustrates that the proposed mathematical model is useful for reducing the vibration localization of a mistuned bladed disk system. The intentional mistuning is used to reduce some power of the bladed disk. Then the level of the forced response is less than the tuned bladed disk system.

The power will be less than the tuned bladed disk system, and it means that

$$\begin{array}{}\text{(24)}& \left\{\begin{array}{l}W=\mathit{\pi }N{f}_k{A}_m+\mathit{\pi }N{f}_k\mathit{\delta }\\ W_{\mathrm{1}}=\mathit{\pi }N{f}_k{A}_m\\ W<W_{\mathrm{1}}\end{array}\right.;\end{array}$$

$${\mathit{\omega }}_m=k\mathit{\omega }\text{and}k=m\mathrm{and}\mathit{\delta }<\mathrm{0}.$$

From Eq. (24), we can get that we can adjust the intentional mistuning parameter for obtaining a small resonance in the bladed disk system. Based on the proposed mathematical model, the power will be less than the tuned bladed disk system, and this shows that the proposed mathematical model is valid for reducing the vibration localization of the mistuned bladed disk system. This is a vital value for the dynamic characteristics of the intentional mistuning bladed disk.

When the mistuning parameter is

$$\begin{array}{}\text{(25)}& \mathit{\delta }>\mathrm{0},\end{array}$$

the power will exceed the tuned bladed disk system, and it means that

$$\begin{array}{}\text{(26)}& \left\{\begin{array}{l}W=\mathit{\pi }N{f}_k{A}_m+\mathit{\pi }N{f}_k\mathit{\delta }\\ W_{\mathrm{1}}=\mathit{\pi }N{f}_k{A}_m\\ W>W_{\mathrm{1}}\end{array}\right.,\end{array}$$

$${\mathit{\omega }}_m=k\mathit{\omega }\text{and}k=m\text{and}\mathit{\delta }>\mathrm{0}$$

From Eq. (26), we can get that if we want to avoid a larger resonance in a bladed disk and we can adjust the intentional mistuning parameter.

When the mistuning parameter is

$$\begin{array}{}\text{(27)}& \mathit{\delta }=\mathrm{0},\end{array}$$

the power will be equal to the tuned bladed disk, and it means that

$$\begin{array}{}\text{(28)}& \left\{\begin{array}{l}W=\mathit{\pi }N{f}_k{A}_m,\\ W_{\mathrm{1}}=\mathit{\pi }N{f}_k{A}_m\\ W=W_{\mathrm{1}},\end{array}\right.,{\mathit{\omega }}_m=k\mathit{\omega }\text{and}k=m\mathrm{and}\mathit{\delta }=\mathrm{0}.\end{array}$$

From the discussion, we can design the intentional mistuning parameter to get a different target.

In this part, in order to illustrate the validity of the proposed model, we can suppose the intentional mistuning parameter $\mathit{\delta }=-\mathrm{0.01}$ and A_m=1. Based on the proposed model, Eq. (24) in this paper, we can get the power of the intentional bladed disk.

When the intentional mistuning parameter $\mathit{\delta }=-\mathrm{0.01}$ and A_m=1, the power of the intentional bladed disk decreases by 1 % compared with the tuned system. Based on the proposed mathematical model, in some situations, we can get the ideal power by adjusting the intentional mistuning parameters. It suggests that we can change deliberate detuning parameters to get the aim of reducing the forced response. First, the main dominant mode shape of the bladed system is calculated under its own rated conditions. Second, the dominant mode shape obtained in the first step is brought into the theoretical model of active detuned resonance proposed in this paper. From the work done in a single cycle, according to the needs of your own working conditions, you can choose the theoretical design of your own corresponding parameters.

Many methods are used to reduce forced response localization. One of the methods is using piezoelectric networking to reduce vibration localization (Zhang et al., 2003; Tang and Wang, 2003; Yu et al., 2006). Previous studies show that intentional mistuning patterns can be used to reduce the vibration localization of bladed disks. However, the determination of the resonance mechanism of the intentional mistuning bladed disk system is still an unsolved issue. In this paper, a novel mathematical model of the resonance of the intentional mistuning bladed disk system is established. Based on the proposed novel model, the vibration localization can be adjusted by using intentional mistuning parameters.

6 Conclusions

In this paper, the determination of the critical resonance mechanism of the intentional mistuning bladed disk system is studied. Many new conclusions are obtained as follows.

1. A novel mathematical model of the resonance of the intentional mistuning bladed disk system is established based on mechanisms of the tuned system.

2. The method of the mechanical power of the rotating blade for one cycle is used to obtain the resonance condition.

3. The results suggest that we can obtain the ideal results by adjusting the intentional mistuning parameter. This is a vital value for the design of the intentional bladed disk system.

The above findings of this paper will give some advice for the design of an intentional mistuning bladed disk and provide guidance for the dynamic vibration control of the mistuned bladed disk.