Structural analysis of traditional Chinese puzzle boxes

A puzzle box (also known as a trick box) is a box that may be opened by solving some obstacles originally designed by the manufacturer. Some puzzle boxes may be opened with a few moves, while others call for problem-solving and decision-making skills to unlock all the blocks inside the box. Puzzle boxes have existed throughout the ancient ages of the east and west, and their popularity has grown significantly since the 17th century. Chinese puzzle boxes are classified into four types based on the first movement during the initial opening stage: whole-box rotation, mechanical-member sliding, mechanical-member rotation, and pin removal. Since the topological structure of the puzzle box changes during the opening process, it falls under the category of the variable topology mechanism. This study proposes a graphical method for systematically analyzing traditional Chinese puzzle boxes. It discusses the structural characteristics of puzzle boxes and applies line graphs to explain the variable topological structures involved in the opening process. The paper presents four different types of puzzle boxes as examples of applying the proposed method and illustrating the opening processes of the puzzle boxes. Traditional Chinese puzzle boxes are diverse and creative, displaying the skilled craftsmanship of ancient artisans. A series of puzzle boxed can be further systematically synthesized through the atlas of line graphs and generalized chains.

1 Introduction

Most families in ancient China kept their personal belongings in shoebox-sized boxes to prevent them from being tampered with by children or unrelated persons. In some regions, when a bride was getting married, she had to prepare a wooden box as part of her dowry to store her jewelry, dressing supplies, and other essential personal items. Some people used these boxes to store important documents, such as accounting books and deeds. Figure 1 depicts one way of locking such boxes using additional metal locks (Rasmussen et al., 2021). However, adding metal locks to wooden boxes was relatively expensive. Therefore, some skilled artisans devised ingenious and creative ways to make wooden boxes with an integrated locking function. In addition to saving money on metal locks, the box owner no longer had to worry about key storage. In response to everyday use and practical needs, Chinese artisans created a variety of shoebox-sized wooden puzzle boxes, usually with a sliding cover plate, and some with drawers or additional special barriers. Such boxes are equipped with mortise-and-tenon work and pins. In some cases, pendulum bobs are also used in puzzle boxes to become obstacles that prevent the boxes from being opened. Combining one or more of these approaches, the mechanism inside the wooden box alone may easily “lock” the puzzle box in place.

Figure 1 Box with an additional metal lock.

Puzzle boxes have a long history in China, but their development and application are hardly documented in ancient literature. Mechanical puzzles were not introduced until the early 19th century to distinguish them from riddles, crosswords, and number puzzles, which did not require objects. Mechanical puzzles were defined as single objects or groups of objects requiring logic, reasoning, judgment, luck, and even the ability to operate and find solutions independently. Since a puzzle box was a subtopic of the mechanical puzzle game, most research on the puzzle box was spread in books about mechanical puzzle game research. Hoffman detailed a variety of 19th-century puzzles and toys, illustrating and exploring the design principles and the puzzle-solving process. In his book, Hoffman presented several puzzle box models designed with friction, spiral, pins, and mortise and tenon (Hoffman, 1893). Slocum was a well-known puzzle collector and historian who had amassed a collection of over 18 000 puzzle-related objects. Examining the puzzle-solving methods and processes, he grouped mechanical puzzles into six categories: combination games, disassembly games, solid interlocking games, unlocking games, sequential games, and puzzle containers (Slocum and Botermans, 1986). Rasmussen et al. (2021) dedicated much of their time to collecting and studying traditional Chinese puzzles and artifacts and exploring the historical development and origins of various traditional puzzles, the interactions and influences between China and the west, and the global distribution of mechanical puzzles. They sorted the puzzles into two categories based on whether they had practical functions. The first category was purely for entertainment and intellectual challenges, such as the tangram, Chinese ring puzzle, burr puzzle, and Klotski (Rasmussen et al., 2021); the second category was for practical and intellectually challenging objects commonly used in daily life, such as cleverly designed and difficult-to-open puzzle boxes and locks; useful and decorative puzzle furniture and rings; and functional puzzle containers, such as fair mugs (a mug with a special trick design in ancient China) and reverse-pouring teapots (Rasmussen et al., 2021). Hajek introduced the flourishing development of puzzle boxes in England, Japan, and Switzerland during the 20th and 21st centuries. He also discussed the mechanical construction, design principles, and opening processes of puzzle boxes in these regions (Hajek, 2021).

The scarce literature on traditional Chinese puzzle boxes resulted in a lack of topological structure analysis for puzzle boxes. Since the puzzle box is a variable topology mechanism, its topology changes in the opening process, making it difficult to conduct a structural analysis. The goal of this study is to analyze traditional Chinese puzzle boxes systematically. This study explores the characteristics of the puzzle boxes first. It presents a set of illustrations that can be used to analyze the structure of each puzzle box. There are four types of puzzle boxes that are provided as examples to demonstrate the structural changes in the opening processes.

2 Characteristics of puzzle boxes

Puzzle boxes are made of a special combination of mechanical members and joints that can block the movement of the cover plate, end plate, bottom plate, rotation plate, pins, and other parts. The puzzle box can only be opened by sliding or rotating all mechanical members according to certain instructions. A mechanical member is a basic element that creates a mechanism and a machine, acting as an object with resistance. It can also serve as a rigid member, a flexible member, or a compression member. In particular, a mechanical member of a puzzle box is mainly composed of rigid members. Rigid members do not deform when in use and are classified into various types: cover plates, end plates, bottom plates, rotation plates, and pins. Meanwhile, a single mechanical member can only be a tool, such as a screwdriver, wrench, hammer, or other similar item. Mechanical members must be combined with precision to perform more complex tasks and obtain increased functionality. A “joint” or “pair” is the way mechanical members are connected. The motion relationship of two mechanical members is defined when their combination is determined (Yan, 1998; Hsiao and Yan, 2014).

Traditional Chinese puzzle boxes were built with a variety of ingenuity and creative approaches to achieve a range of unique opening methods and different design styles. Figure 2a depicts a puzzle box designed with a bob. The figure shows that the puzzle box contains three mechanical members: the case, a bob, and a cover plate. The cover plate has a curved groove on one end just above the bob's top half, making it impossible for the cover plate to move, thereby locking the wooden box entirely. The first step in opening the box is to rotate the puzzle box by about 90°. The bob will only slightly rotate due to the center of gravity so that the upper part of the bob is no longer against the cover plate. As shown in Fig. 2b1–2b3, the cover plate can then be unfixed, moved to the right, and moved out of the case to open the puzzle box.

Figure 2 Puzzle box with a bob.

3 Graphic representations

The graph theory defines a graph as a set of connected vertices and edges. Graphic representations can be used to perform the structural analysis and structural synthesis of a mechanism. This study applies graphical concepts to analyze the structure of the puzzle box (Tsai, 2001; Kuo, 2008).

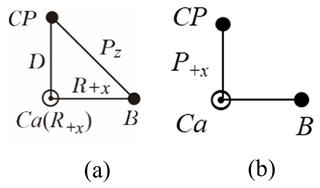
A right-handed Cartesian coordinate system is used to represent the topology of a puzzle box and describe the relationship between the movements of the mechanical members in the puzzle box. In this coordinate system, the direction of the cover plate moving out of the case is defined as the positive x-axis direction. Meanwhile, the upward direction of the box is defined as the positive y-axis direction. The positive z axis is defined by the right-hand rule as shown in Fig. 2a (Shi et al., 2017).

Based on this defined coordinate system, a puzzle box's mechanical members and joints could be represented by vertices and edges, respectively. When two mechanical members are connected, the corresponding mechanical members are represented by two vertices, and the combination of the two mechanical members is represented by an edge.

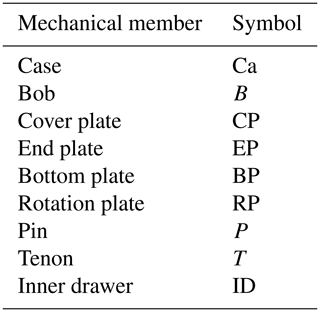
As shown in Table 1, the mechanical members of the puzzle box are represented by vertices and their names, and edges represent the joints of the puzzle box. There is also a subscript below the type name of the joint, which represents the direction of its axis movement (Hsiao, 2017, 2018; Shi et al., 2020). If the motion axis of a rotating joint is parallel to the positive x axis, it is marked as R_+x; if the motion axis of a sliding joint is parallel to the negative y axis, it is marked as P_−y and so on. On the other hand, if the positive and negative directions of the motion axes do not affect the movement, the positive and negative symbols can be ignored and not marked. If the two mechanical members are fixed to each other, it is a direct contact joint, which is marked as D. It can be ignored if the two mechanical members are connected without relative movement. As shown in Fig. 2, the first step in opening the puzzle box is to rotate the entire box. Since the case, Ca, rotates directly in the positive x-axis direction, it can be represented as Ca(R_+x). The bob, B, and the cover plate, CP, are connected to the case with joint R_+x and joint D, respectively. The bob, B, is connected to the cover plate, CP, with joint P_+z. Figure 3a shows the illustration of the first step in the opening process, i.e., locking the case at the starting stage. The second step to open the puzzle box is to move the cover plate CP out of the case in the positive x-axis direction. The cover plate is connected to the case with joint P_+x. At this point, the bob and the case do not have relative movement and can be ignored without marking. Since the bob and the cover plate are not connected, drawing the edges is unnecessary. Figure 3b shows the graphical illustration for this movement.

Figure 3 Graphic representations of a puzzle box with a bob.

Table 1 Illustrations of the mechanical members.

4 Structural analysis

Traditional Chinese puzzle boxes are created with diverse ingenuity and creativity to form a series of puzzle boxes that open in many exciting ways, are integrated with mortise-and-tenon work, and have varied design styles. To conclude the classification of obstacles of available objects, the kinds of obstacles of puzzle boxes are the bob, groove, geometric constraint, and pin. Corresponding to the first step of opening box, these types of traditional Chinese puzzle boxes involves four types of motion, which are explained below: whole-box rotation, mechanical-member sliding, mechanical-member rotation, and pin removal.

4.1 Type 1: whole-box rotation

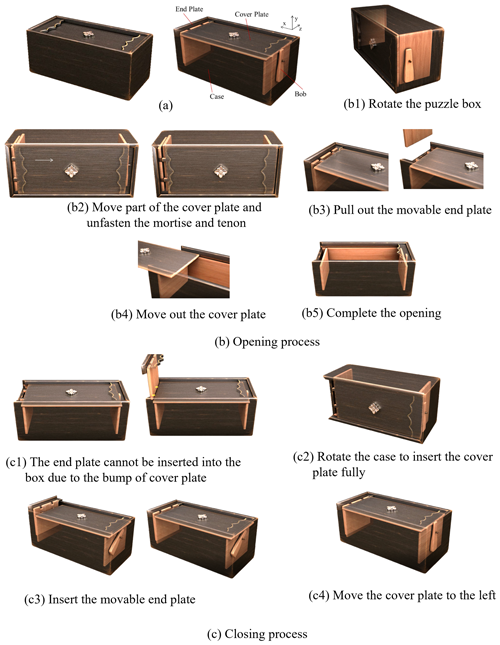
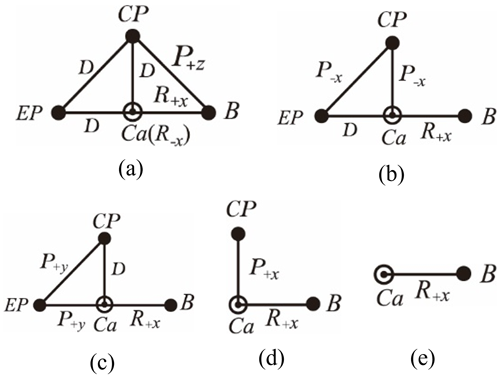
Figure 4 depicts a puzzle box with combined bob and mortise-and-tenon work, as well as four mechanical members: case (Ca), bob (B), cover plate (CP), and movable end plate (EP). As shown in Fig. 4a, one end of the cover plate and the movable end plate are fixedly joined by mortise and tenon, while the other end rests against the bob's top half, immobilizing the cover plate and locking the entire wooden box in place. This obstacle of the puzzle box is the bob. Figure 4b1 illustrates the corresponding 3D model for this step. The corresponding graphic representation is shown in Fig. 5a.

Figure 4 A 3D model of a whole-box rotation puzzle box.

Figure 5 Graphic representations of a whole-box rotation puzzle box.

The first step to open the box is to rotate it 90° toward the negative x axis so that the bob is no longer against the cover plate. At this point, the cover plate, the movable end plate, and the case are fixed to each other, while the bob is connected to the case through joint R_−x. The cover plate and the bob are not in contact. For the second step, the cover plate must be slightly moved along the negative x axis to release the mortise and tenon. Figure 4b1 and b2 illustrate the corresponding 3D models for these two steps. According to the variable topological structure, the joint is incident to member B, and member CP is inexistent; thus, the line between B and CP is canceled. The graphic representation corresponds to Fig. 5b. The third step requires pulling out the movable end plate along the positive y axis and pulling out the cover plate completely along the positive x axis to fully open the puzzle box as shown in Fig. 4b3. The graphic representations are shown in Fig. 5c. By removing the cover plate, the corresponding 3D model is shown in Fig. 4b4, and the graphic representations are shown in Fig. 5d. Finally, Fig. 4b5 shows the complete opening situation, and the graphic representations of the topological structure are shown in Fig. 5e.

To close the box, if the cover plate is inserted directly, it cannot completely slide to the deepest location above the box due to bob obstruction, and the bump of the cover plate makes inserting the movable end plate difficult as shown in Fig. 4c1. Therefore, the puzzle box must be rotated by 90° before inserting the cover plate and movable end plate as shown in Fig. 4c2. And thus, the end plate can be inserted, and the cover plate can be moved to the left to finish the process of closing the puzzle box.

4.2 Type 2: mechanical-member sliding

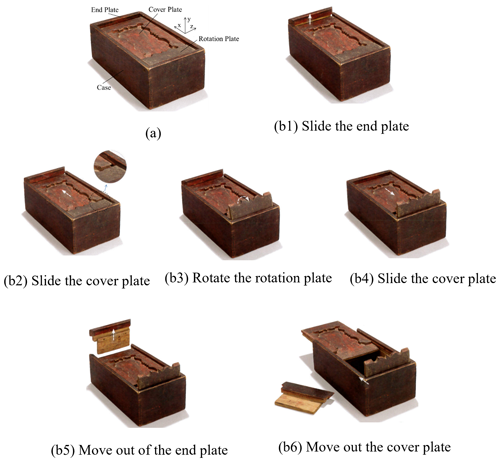
Traditional Chinese puzzle boxes, which use sliding mechanical members as the first step of opening, come in various forms. Different mechanisms and mortise-and-tenon designs are used to create various opening steps. Figure 6a depicts a puzzle box requiring sliding the end plate first, which includes four mechanical members: case (Ca), end plate (EP), cover plate (CP), and rotation plate (RP). This obstacle of the puzzle box is a groove.

Figure 6 Mechanical-member sliding puzzle box.

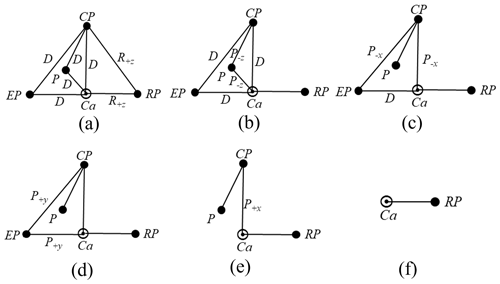
The first step to open a mechanical-member sliding puzzle box is to slide the end plate partly along the positive y axis to free up space on the left side before moving the cover plate partly along the positive x axis as shown in Fig. 6b1 and b2. The graphic representations of topological structures are shown in Fig. 7a–b. With the opening process, the joint incident to the rotation plate and the cover plate changes from direct contact to a prismatic joint, and then the rotation plate and the cover plate are separate. The prismatic joint incident to the cover plate and the end plate changes the sliding directions from the positive y axis to the positive x direction, and then the prismatic joint becomes direct contact. The prismatic joint between the end plate and the case transforms into direct contact owing to the groove constraint.

Due to the movement of the cover plate, the fixation between the cover plate and the rotation plate is released, allowing for the rotation plate to be rotated in the positive z-axis direction while simultaneously freeing up space on the right side so that the cover plate can be moved in the negative x-axis direction as shown in Fig. 6b3 and b4. The graphic representations of topological structures are shown in Fig. 7c–d. The joint incident to the rotation plate and the case transforms direct contact into a rotation joint after sliding the cover plate. Then, the rotation plate and the case still connect but have no relative motion. Next, the end plate can be pulled out entirely along the positive y-axis direction as shown in Fig. 6b5. The last step to completely open the puzzle box is by moving out the cover plate along the positive x axis as shown in Fig. 6b6. The graphic representations of topological structures corresponding to these two steps are shown in Fig. 7e–f.

Figure 7 Graphic representation of a mechanical-member sliding puzzle box.

4.3 Type 3: mechanical-member rotation

Several types of traditional Chinese puzzle boxes open through mechanical-member rotation as the first step. Figure 8a shows a mechanical-member rotation puzzle box that conceals the pin. It contains five mechanical members, including the case (Ca), an end plate (EP), a cover plate (CP), a rotation plate (RP), and a pin (P). A rotation plate and an end plate prevent the cover plate from moving, while a movable pin secures the cover plate to the case. This obstacle of the puzzle box is a geometric constraint.

To open this box, the first step is to rotate the rotation plate in the direction of the positive z axis followed by moving the movable pin slightly in the direction of the negative z axis to move the cover plate in the direction of the negative x axis as shown in Fig. 8b1–b3. Only after releasing the fixed relationship between the cover plate and the end plate can the end plate be moved out along the positive y axis as shown in Fig. 8b4. The final step involves moving out the whole cover plate along the positive x-axis direction as shown in Fig. 8b5. Figure 8b6 shows the complete opening situation. The graphic representations of the topological structure in each opening step are presented in Fig. 9.

Figure 8 Mechanical-member rotation puzzle box.

Figure 9 Graphic representation of mechanical a member rotation puzzle box.

4.4 Type 4: pin removal

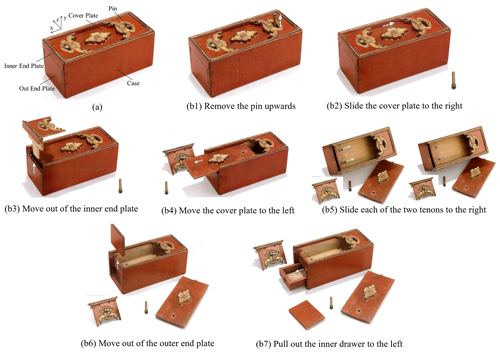
Pin-removal puzzle boxes use movable pins as the first opening step and usually require other mortise-and-tenon work, making the opening process more complicated. Figure 10a shows a pin-removal puzzle box with a hidden drawer. The mechanical members contain a case (Ca), a cover plate (CP), an inner-end plate (IEP), an outer-end plate (OEP), two tenons (T), an inner drawer (ID), and a pin (P). This obstacle of the puzzle box is a pin.

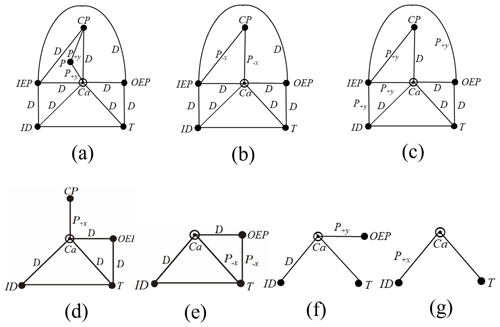
The first step to open this box is to move the pin out along the positive y axis and then move the cover plate partly along the negative x axis before moving the inner-end plate along the positive y axis. The above opening process is shown in Fig. 10b1–b3. This is then followed by moving the cover plate out entirely along the positive x-axis direction so that the upper space of the puzzle box would appear as shown in Fig. 10b4. Afterward, the two tenons along the negative x axis must be moved to release the fixed mortise-and-tenon relationship between the tenons and the outer-end plate as shown in Fig. 10b5. This way, the outer-end plate can be moved along the positive y axis as shown in Fig. 10b6. Since the inner drawer can only be found after the outer-end plate is removed, the inner drawer must finally be moved along the positive x-axis direction as shown in Fig. 10b7. A total of seven steps are required to open the box altogether. The graphic representations of the topological structure in each opening step are presented in Fig. 11.

Figure 10 Pin-removal puzzle box.

Figure 11 Graphic representations of a pin-removal puzzle box.

5 Conclusions

Traditional puzzle boxes have been popularly used for both practical and educational purposes for centuries. In addition, these puzzle boxes are widely used to store important personal items and documents. Ancient artisans continued researching, exploring, and creating various puzzle boxes with variable topology mechanisms and ingenious mechanical structure and design principles. These features are a product of ancient artisans' repeated designs and manufacturing, accumulated working experiences, and creative ideas. Traditional Chinese puzzle boxes can be classified into four types based on the first step of opening: whole-box rotation, mechanical-member sliding, mechanical-member rotation, and pin removal. The kinds of obstacles included the bob, groove, geometric constraint, and pin by observing available puzzle boxes. The illustrations in this study utilize the four puzzle types as examples to describe the proposed method and to systematically analyze traditional Chinese puzzle boxes. The method using graphic representations to represent the variable topological structures and the opening process is presented. The graphic representations help understand the relative motions between mechanical members and the operating function. This proposed method is an effective tool for demonstrating the structural changes in the puzzle boxes during the opening processes. It can be used to perform a structural analysis of other variable topologies and is regarded as a hint for unlocking the puzzle boxes.

Further, the proposed method can be used to construct more new puzzle boxes by assigning different joint types with the same degree of freedom. The atlas of line graphs can transform into the atlas of generalized chains through graph theory. A series of puzzle boxes can be systematically synthesized based on the creative design methodology. Therefore, the proposed method is useful for understanding the mechanical structure hidden in the puzzle boxes and the solution to opening the boxes as well as creating a new one.